Abstract

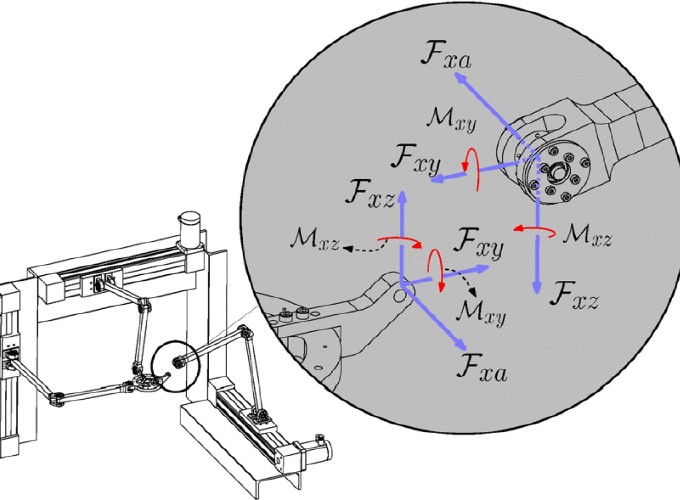
This paper presents a new approach for the dynamic modeling of a 3-degree-of-freedom parallel manipulator, called the Tripteron. The reasoning used in this paper is based on a judicious concept in detaching the whole mechanism into several subsystems and consecutive synergies between kinematic analysis, Lagrangian and Newtonian approaches. In this regard, the Tripteron is made equivalent to four subsystems and the equations of kinematic constraints are derived for all subsystems. Afterwards upon resorting to Lagrangian approach and blending it with the latter kinematic relations, the dynamic model of each leg in task space is obtained. The dynamic model of the end-effector is written in virtue of Newton-Eulers approach where yields to three differential equations. Finally, the problem leads to a system of 12 equations and 12 unknowns. For the sake of comparison, the results are put into contrast by the one obtained with a dynamic analyser software. The results obtained by both approaches are coherent which affirms the correctness of the proposed algorithm